Eclipse 安装Gradle插件
本文共 702 字,大约阅读时间需要 2 分钟。
前言说明:Gradle也是一种通过依赖配置减少jar下载的方式,类似于Maven的原理。
环境要求:环境jdk一定要是1.7以上,否则插件安装会失败。
项目地址:
1.离线安装方式
第一步:下载Gradle>
第二步:解压gradle-2.5,
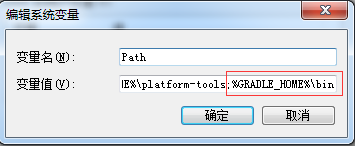
配置环境变量:GRADLE_HOME

path添加;%GRADLE_HOME%\bin
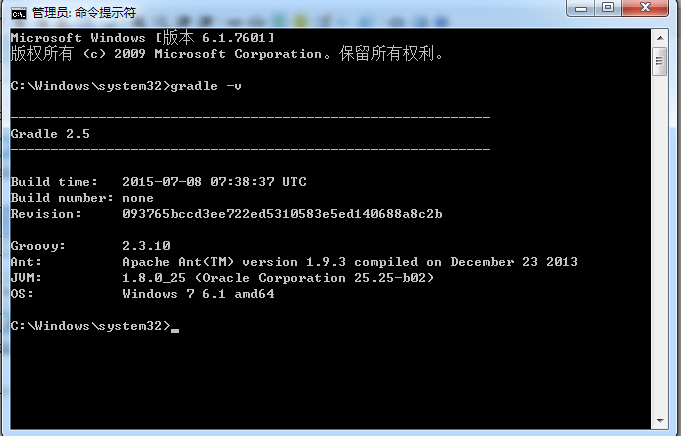
第三步,查看是否安装成功:
以管理员身份运行"命令提示符",输入:gradle -v
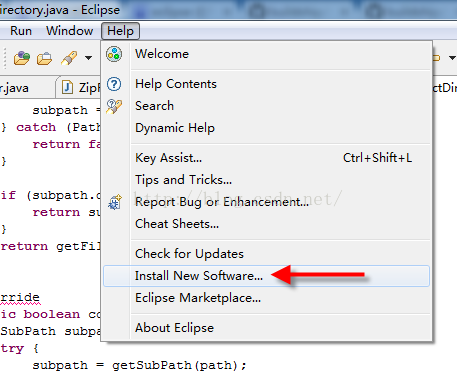
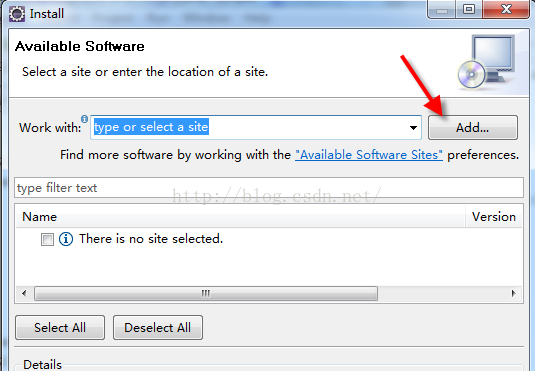
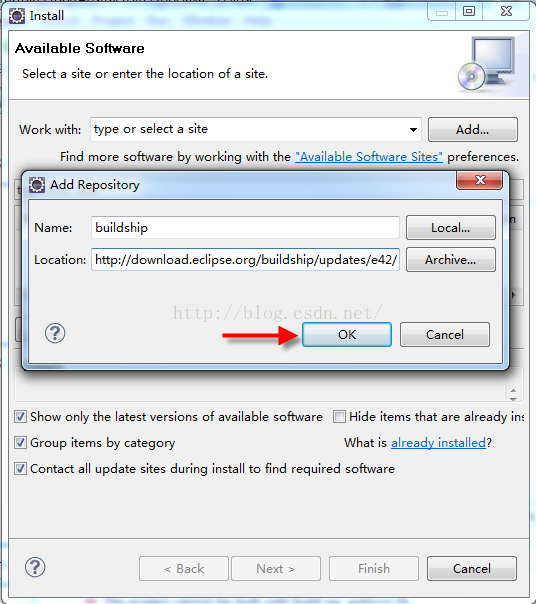
第四步:打开eclipse>Help>Install new software>输入http://dist.springsource.com/release/TOOLS/gradle
next的过程中,如下图步骤的时候一定要选择第三项,并且全部勾上,然后一直next;
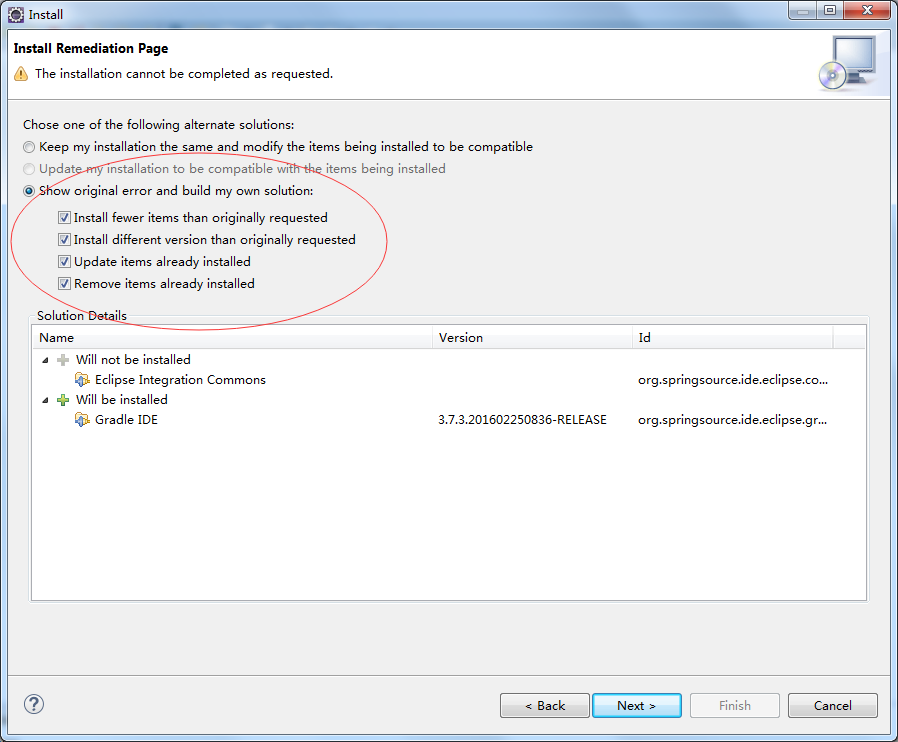
安装成功后提示重启eclipse;
第五步:eclipse安装gradle插件后,导入gradle项目报错,右键项目-->Gradle-->Refresh All就OK了。
2.Eclipse在线更新方式
(1)在线安装地址指南:
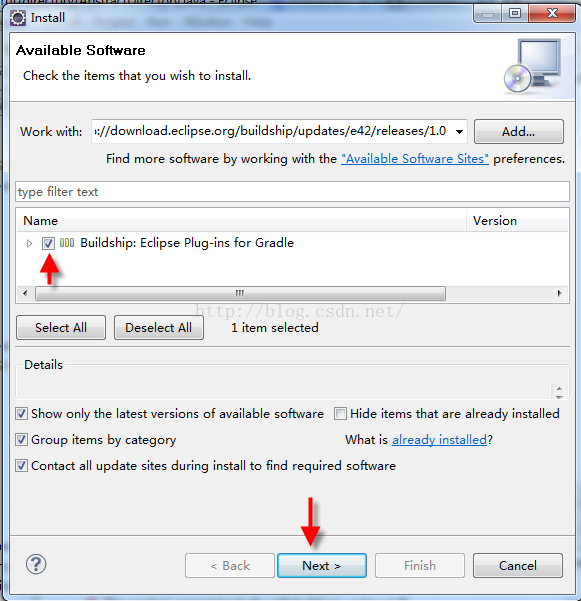
找到对应的eclipse版本拷贝链接。(2)打开eclipse,依次按以下步骤操作
3.Eclipse Marketplace方式
eclipse中依次打开“Help”–》“Eclipse Marketplace”,在搜索栏中输入“buildship”进行搜索;
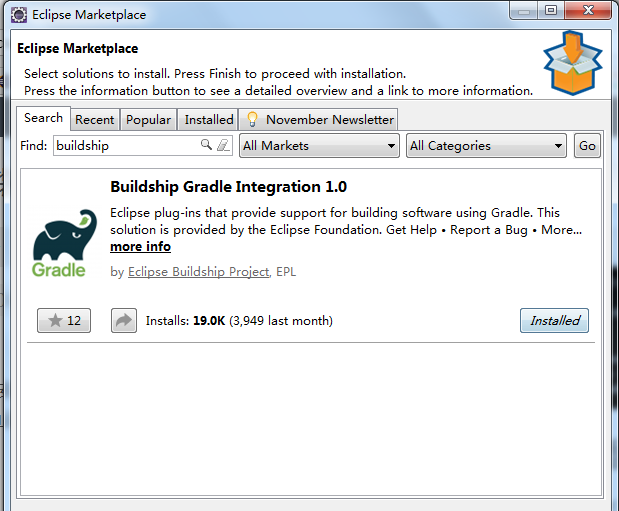
2.点击install,等待安装完成,按照提示重启eclipse即可。
3.安装成功后,可以在Window–>Show View–>Others…中和File–>New–> Other…中看到增加的Gradle选项。
你可能感兴趣的文章
MySQL高级配置
查看>>
MySQL高频面试题
查看>>
MySQL高频面试题的灵魂拷问
查看>>
MySQL(1)的使用 | SQL
查看>>
MySQL(2)DDL详解
查看>>
MySQL(3)DML详解
查看>>
MySQL(4)运算符 | 关联查询详解
查看>>
MySQL(5)条件查询 | 单行函数 | 事务详解
查看>>
Mysql,group by分组查询、order by排序查询、join连接查询、union联合查询
查看>>
Mysql,sql文件导入和导出
查看>>
MYSQL:int类型升级到bigint,对PHP开发语言影响
查看>>
Mysql:mysql 5.X 报错 ERROR 1193 (HY000): Unknown system variable ‘validate_password_length‘
查看>>
MySQL:MySQL执行一条SQL查询语句的执行过程
查看>>
Mysql:SQL性能分析
查看>>
mysql:SQL按时间查询方法总结
查看>>
MySQL:什么样的字段适合加索引?什么样的字段不适合加索引
查看>>
MySQL:判断逗号分隔的字符串中是否包含某个字符串
查看>>
MySQL:某个ip连接mysql失败次数过多,导致ip锁定
查看>>
MySQL:索引失效场景总结
查看>>
Mysql:避免重复的插入数据方法汇总
查看>>